When two talking heads are better than two flips of a coin: Social interaction helps team performance

Put a few people together on a team and you get teamwork, which Wikipedia lauds as “a means of assuring quality and safety in the delivery of services” in at least some domains.

Put a few people together on a team and you get group think, which Wikipedia censures as “actively suppressing dissenting viewpoints”, citing also the risk that group members “isolate themselves from outside influences.”

Put two heads together and they are better than one. Put two heads together and they engage in group think or they do worse than the better head on its own. Which is it? Are two heads better than one, and if so, are there boundary conditions to this beneficial effects?

A recent article in the Psychonomic Bulletin and Review was aimed at some of those issues. Researchers Allison Brennan and Jim Enns were particularly interested in whether a benefit that might accrue from collaboration between two people arises from purely statistical facilitation or an additional boost from social interaction.

To understand this distinction, it is helpful to consider a few known findings. For example, it is known that when a task involves noisy visual signals, pairs of people who communicate their confidence to each other perform better than individuals. Specifically, if two displays such as those in the figure below are shown successively for brief periods, then two people are better at picking the patch with slightly greater contrast (top left in the first display) than individuals on their own.

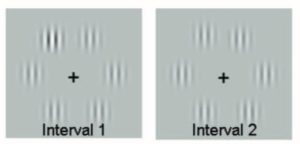
So far, so good.

Except that it is also known that the average of multiple estimates from the same person can outperform any single best estimate by that person. And except that in the noisy visual signal task in the above figure, the same effect is obtained if instead of using a pair’s joint response, the response of the more confident individual is used.

The fact that the superiority of joint performance can be mimicked by the same person (by providing a judgment repeatedly) or by people that do not work together (by picking the more confident response) suggests that social interaction may not be required for two heads to be better than one. What we may be observing instead is a simple statistical phenomenon: For example, two responses from the same person may be better than each on its own if the errors that are associated with each response cancel each other out.

How might one differentiate between a purely statistical phenomenon and “true” social facilitation that somehow “adds value” to performance?

Brennan and Enns used a mathematical tool to examine this question that, despite its sophistication, is based on a very simple idea, namely that of statistical independence. In a nutshell, independence is present when two events do not affect each other in any way: for example, the toss of a coin in Romania today will not affect whether a penny tossed in England tomorrow will come up heads or tails. When independence is present, the probability of occurrence of both events can be computed from knowledge of each event’s probabilities by simple multiplication. The probability of the Romanian and English coins both coming up heads is therefore .5 × .5 = .25.

Things are very different when two events are not independent: If one connects one coin to another with sticky tape (which allows some movement but does favor both coins coming up in the same direction), then if one comes up heads the probability of the other one following suit is nowhere near .5, and hence the probability of both being heads is not equal to .25.

It follows that by observing the probability of many joint events, we can infer whether we are dealing with two independent coins or a pair that a sneaky experimenter has joined by sticky tape. For now, let’s just replace the coin in the above example with a participant in an experiment, and the logic becomes apparent: if people’s interactions go beyond what one might expect on the basis of statistical combination, then we should not observe independence—that is, the observed team performance should not be predicable from individual performance by application of something analogous to simple multiplication (people are different from coins, so the way in which independence manifests itself is different from that for coins, but the idea is the same).

Using that logic of inference, Brennan and Enns observed performance in a visual search task under various different combinations of individual and team performance. The figure below shows their display, consisting of more than 80 objects and four targets, some number of which might be present in the shelf.

The task is to search for the targets in the display and determine how many of the 4 are present (the answer could be 0, 1, or 2).

Try it now, and if you can stick your head together with someone else, all the better.

In the study by Brennan and Enns, people performed this visual search task twice, once on their own and once with their partnered participant. On each occasion participants went through many trials. Because the task was relatively simple, emphasis was on the time people took to report how many targets were present: On most trials, the correct number was reported, so accuracy was of lesser concern than response time. Response times were also required because the mathematical tool that was used to determine whether or not social interaction benefited team performance was based on response latencies.

Interpretation of the results focused on whether or not team performance could be predicted from the same participants’ individual performance on the basis of independence—that is, were the “coins” independent or were they joined at the hip, so to speak, when performing the task?

Brennan and Enns found that the benefit of team performance was a clear result of social interaction. Two heads were better than one, not just because of the statistical facilitation of response times, but because being together added value to the team’s performance.

Moreover, buttressing this principal conclusion, the magnitude of the team advantage was strongly associated with the degree of social affiliation between pair members: Participants were recruited as pairs of friends but of course the depth of friendship differed between pairs and was measured through a questionnaire. The greater the friendship, the better the two heads did relative to just one person on their own.

Unlike previous demonstrations of superior team performance, the study by Brennan and Enns was the first to pin down by rigorous mathematical means that there was more to the team advantage than statistical facilitation.

So if you haven’t found the targets in the above picture yet, call your best friend to come over and try again.